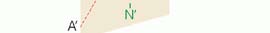optique/cours/chap2.html#x1-92005
http://www.uel-pcsm.education.fr/consultation/reference/physique/optigeo/
menumodule/menuthemes/menu_reduit.html
http://www.univ-Lemans.fr/enseignements/physique/02/optigeo/
http://fr.wikipedia.org/wiki/Optique
http://cours.cegep-st-jerome.qc.ca/203-301-r.f/prtie2/chap6/default.htm
Activity Introduction
Let us take a glass of water and dip a spoon into it. What seems to happen? The
spoon looks like it’s bending where it meets the water. The submerged part of
the spoon makes the glass look shallower than it is, and we can see the reflection
of the part of the spoon that is above water. All of these elements demonstrate
the way the light’s behavior changes as it goes through the water’s surface, i.e.,
the reflection on the surface can only be the image of the part of the spoon that is
emerging from the water’s surface that is acting as a reflective material. We will
be explaining all of these observations in this chapter.

African Virtual University
Formative Evaluation
1- Draw a commented (annotated?) sketch that highlights the Snell-Descartes
Laws; (but this sketch has already been given in the text earlier)
2- Recall the physical meaning of each element of the Snell-Descartes Laws;
3- If light passes from one medium (1) to another medium (2) with n < n , which
1
2
inequality describes the relation between angles i and i ?
1
2
a) i < i
1
2
b) i > i
1
2
c) i = i
1
2
Check off the right answer(s).
4- In that case, how is the ray refracted with respect to the normal? The refracted
ray:
a) moves closer to the normal
b) moves away from the normal
c) stays in the same line as the incident ray
Check off the right answer(s).
5- Therefore, when the angle of incidence increases, the angle of the refracted
ray will reach a maximum value.
a. True
b. False
6- If light passes from one medium (1) to another medium (2) with n > n , which
1
2
inequality describes the relation between angles i and i ? You may use the
1
2
answer to question 3 by applying the opposite light rule.
a) i < i
1
2
b) i > i
1
2
c) i = i
1
2
Check off the right answer(s).
7- In that case, how is the ray refracted with respect to the normal? The refracted
ray:
a) moves closer to the normal
b) moves away from the normal
c) stays in the same line as the incident ray
Check off the right answer(s).

African Virtual University
8- Therefore, when the angle of incidence increases, the angle of the refracted
ray will reach a maximum value. You may use the answer to question 5 by
applying the opposite light rule.
a. True
b. False
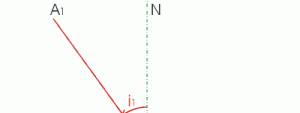
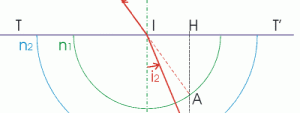
9- Geometrical construction: Recall the definition for the plane of incidence.
10- Recall the definition of the point of incidence I;
11- Take the plane of incidence for a figure plane and draw an incident ray A I,
1
where I is the point of incidence. Draw a circle with center I and radius in
proportion to n . Extending the incident ray will intersect the circle at point A.
1
Take TT’, the line representing the plane tangent by I to the surface boundary
between the two media with indices n and n , with AH being the normal at
1
2
TT’ and passing through A. Draw a circle with center I and radius in propor-
tion to n . Extending AH will intersect the circle proportional to n at point
2
2
A . The refracted ray is IA . Please note that for n > n , the circle with radius
2
2
2
1
n is inside the circle with radius n and the construction is still possible. On
1
2
the contrary, if n < n the uttermost ray that can penetrate into medium with
2
1
index n intersects the circle with radius n at a point B such as the normal
2
1
BH is tangent to the circle of radius n . We have now graphically construed
2
the critical angle l ' = l . Using this method, double-check the answers you
have chosen for questions 3 to 8.
12- Problem 1: A beam of light passes from the air to a liquid; it is deviated by
19º and the angle of incidence is 52º. Select the liquid’s refractive index from
the following values:
a) 0.83
b) 1.45
c) 2.42
d) 1.20
Check off the right answer(s).
13- Problem 2: With respect to the horizontal, in which direction does a diver
at the bottom of the ocean see a sunset? The water’s refractive index is n =
2
1.333 and the air’s is n =1.
1
a) 0°
b) 41.4°
c) 48.6°
d) 90°
Check off the right answer(s).

African Virtual University
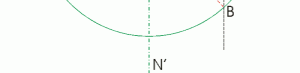
14- Problem 3: When a diver at the bottom of a lake looks up to a perfectly calm
surface, it looks dark to her, except in a circular area that is exactly above her.
Determine the angle implied by this illuminated area. The water’s refractive
index is n = 1.333 and the air’s refractive index is n =1.
2
1
a) 41.4°
b) 48.6°
c) 82.8°
d) 97.2°
Check off the right answer(s).
15- Define a plane mirror.
16- The mirror-image A’ of a point A is symmetrical to A in relation to the mir-
ror’s plane.
a. True
b. False
17- A written text, reflected by a mirror is reversed.
a. True
b. False
18- We place two mirrors face to face, each one with a length of 1.5 m. The dis-
tance between them is 15 cm. A ray of light falls onto the edge of one of the
mirrors, with an angle of incidence of 15º. How many times will the ray be
reflected before it makes it to the other edge?
To solve this problem, draw a sketch that represents the reflection angles
and then determine the horizontal distance that the ray travels between two
reflections. There will be:
a) 15 reflections
b) 30 reflections
c) 37 reflections
d) 74 reflections
Check off the right answer(s).

African Virtual University
19- Problem 5: Two plane mirrors are parallel to and 20 cm away from each other.
A glowing spot is placed between them, 5 cm away from the first mirror. De-
termine the distances of the three nearest images that appear in each mirror
in relation to the other. For the first mirror, the images are at:
For the first mirror
For the second mirror
a-) 5 cm
a-) 5 cm
b-) 10 cm
b-) 10 cm
c-) 15 cm
c-) 15 cm
d-) 20 cm
d-) 20 cm
e-) 25 cm
e-) 25 cm
f-) 35 cm
f-) 35 cm
g-) 40 cm.
g-) 40 cm
20- Teamwork:
Required material: Mirror, large sheet of paper, needles.
Procedure: Place the large sheet of paper on a table and place the mirror on
top, taking care to secure the mirror orthogonally to the table. Trace along the
bottom of the mirror. One group will plant some needles vertically onto the sheet
of paper, taking care to line them up at equal distances and push them in at the
same height. A second group will plant some needles vertically onto the sheet of
paper, in the same way as the first group and taking care to line them up with the
images of the needles planted by the first group.
Double-check that the needles planted by the second group are perfectly lined up
with the needles planted by the first group.
Take down the assembly and keep track of the holes from the needles that were
planted. Draw a line (D ) through the positions of the needles planted by the first
1
group, and do the same with a line (D ) for the second group. Make sure the two
2
lines intersect at a point I that is on the line that was traced along the bottom
of the mirror. Draw a line (D) perpendicular to the mirror starting from point I.
Make sure that the lines D and D are symmetrical to D. This demonstrates the
1
2
law of reflection.




African Virtual University
Answer Key
1-) and
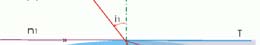
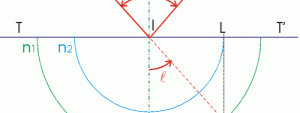
2-) Critical Refractive Angle
If light travels from a less refractive medium (1) to a more refractive medium (2),
as in if n < n , according to DESCARTES’ 3rd law we will have:
1
2
n sin i
sin i
2
2 = n1
1
or,
n1 < n2 ⇒ sin i2 < sin i1
therefore, i2 < i1
Since both angles are acute and have the same sign, any incident ray A I will
1
correspond to a refracted ray IA which gets closer to the normal as it enters
2
the more refractive medium. (The annotations, where applicable must also be
translated into English)
Feedback: Excellent diagram and commentary.
3.
a. Read the question again.
b. Very good answer. The refractive indexes are indeed inversely proportional
to the angle of incidence.
c. Think about the relation between the angle of refraction and the angle of
incidence.

African Virtual University 0
4.
a. Excellent, your answer is correct.
b. Review the lesson on refraction.
c. Carefully read the question again.
5.
a. Good job, a does indeed end up with the maximum incidence skimming
1
value of 90°. The value of a is limited and smaller than 90°.
2
b. Read the question again to understand it better.
6.
a. Good answer, since medium 1 is more refractive than medium 2, we do
indeed obtain i <i
1
2
b. Please try again.
c. You have misread the question
7.
a. Try again
b. Great answer.
c. Review the lesson.
8.
a. This is the wrong choice.
b. Very good answer. When the incident ray’s angle increases, there is indeed
no more refracted ray. There is total internal reflection.
9-10- 11. Geometrical construction
Feedback : Good diagrams and commentary.

African Virtual University
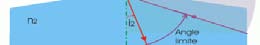
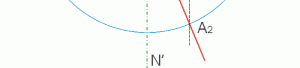
Surface Index Method.
The plane of incidence is used as the figure plane.
Using the point of incidence I as the center, we draw two circles with radii pro-
portional to n and n . Extending the incident ray A1I intersects the circle with
1
2
radius n1 at point A. If TT’ represents the plane that is tangent by I to the surface
separating the two media with indexes n and n , the normal AH it intersects the
1
2
half-circle with radius n at point A2. The refracted ray is IA2.
2
When n > n , the circle with radius n is inside the circle with radius n and the
2
1
1
2
construction is still possible. On the contrary, if n < n the uttermost ray that
2
1
can penetrate into medium with index n intersects the circle with radius n at a
2
1
point B such as the normal BH is tangent to the circle of radius n . We have now
2
graphically construed the critical angle l ' = l .
12.
a. This is the wrong answer.
sin 52°
b. Good answer, as a matter of fact n =
sin (52° −19°)
c. Think about the relation between the refractive index and the angle of
incidence.
d. Read the question once more and then try again.
13.
a. Careful, Try again.
b. The critical angle for refraction is 48.6°. In relation to the horizontal, we
have 90°- 41.4°.
c. Try again
d. Read the question once more and then try again.
14.
a. Take your time to read the question before answering.
b. Try again
c. Try one more time
d. Good answer, the implied angle is indeed equal to double the critical angle
of refraction.



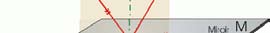


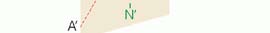

African Virtual University
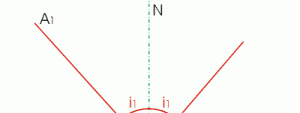
15. Mirror-image
The ray AH, normal to the mirror, doubles back on itself: the image of A, if it
exists, is therefore on the normal.
The reflected ray IR of some incident ray AI is in the plane of incidence AIN which
also includes AH (since AH and IN are parallel, like lines that are perpendicular
to the same plane, and that by definition, A is part of the plane of incidence.
IR’s support meets AH at a point A’. (The annotations, where applicable must
also be translated into English)
(It is important to number the figures are appropriately refer to them in the
text)
In the triangle AIA’, the height IH is also the bisector. The triangle is therefore an
isosceles triangle. Thus, IH is also the median and we get AH = HA’.
This shows that A’ is symmetrical to A with respect to the mirror’s plane, no
matter which incident ray is considered. A’ is the image of A.
16.
a. Good answer.
b. You have misread the question.
17.
a. Good answer
b. You have not thought it through before answering.
18.
a. Try again
b. Careful, you answered too fast. Try again
c. Very good.
d. Please try again, you have misunderstood the question.

African Virtual University
19) For the first mirror : a-e-f. Very good.
For the second mirror: b-d-g. Very good.
Give the following feedback for all other answers : review a mirror’s charac-
teristics.
Self Evaluation
The learners write down the difficulties they encountered while solving the
problems. Following those difficulties, each group will be oriented towards the
Power Point presentations or the interactive animated website at the following
address: http://www.sciences.univ-nantes.fr/physique/enseignement/DeugA/
Physique1/optique/TP-info/index.html. (any equivalent site in English?) Each
group will then debrief the tenured teacher or tutor about their results in an atta-
chment sent by e-mail.
Teacher Guidelines
The tutor will open the chat window, correct all of each group’s answers and
briefings sent in an attachment by e-mail. The appropriate feedback will be sent
back to each group by e-mail and the activity’s general solution will be posted
on the ‘Student’ workspace. The grade received from this activity accounts for
20% of the module evaluation.


African Virtual University
Learning Activity 3
Activity Title
Boundaries and Curved Mirrors
Required Time
8 hours
Specific Objectives
The learner should be able to:
Describe a curved boundary;
•
Describe a curved mirror;
•
Construct the image of a real object placed before a curved boundary
•
Construct the emerging ray that corresponds to an incident ray
Determine a curved boundary’s characteristics using the conjugate equations
Describe the characteristics of an image produced by a curved boundary.
Activity Summary
Through this activity you will learn how to describe a curved boundary and a
curved mirror and also be able to determine their characteristics. With these ele-
ments the conditions for astigmatism, or at least near-sighted astigmatism, will
be defined and the conjugate relations will be established.
You will then have to define the characteristics of a curved boundary followed
by those of a curved mirror. This way you will be able to draw the path of a ray
of light and predict experimental results using the characteristics of a curved
boundary.
Key Concepts
Main Boundary Axis: The diameter of the sphere that is perpendicular to the
dome’s useful surface, also known as the optical axis.
Object Focal Point: The point where the rays coming from the object meet the
optical axis and come out parallel to it.
Image Focal Point: The object rays that are parallel to the optical axis exit the cur-
ved boundary and meet the optical axis at the image focal point. Image Focal

African Virtual University
Plane: Home to the image’s ‘secondary focal points’ like Φ where the cylindri-
2
cal beams parallel toC Φ converge. This plane passes through the image focal
2
point and is orthogonal to the main axis. (These symbols have not been defined.
Which figure is being referred to?)
Magnification: The relation between the dimensions of an image in the frontal
plane in A and the corresponding dimensions of the object in A . (These symbols
2
1
have not been defined. Which figure is being referred to?)
Activity Description
This educational activity consists of gaining a better understanding of the defi-
nitions and reasoning behind the different demonstrations used to establish the
rules and characteristics relative to a curved boundary. You will have to know how
to calculate the curved boundary’s different characteristics using the conjugate
relations. You will also be expected to know how to graphically solve curved
boundary problems.
This learning activity is made up of problems related to curved mirror and boun-
dary characteristics. They are multiple choice questions and the learner will have
to check off the answers that he or she believes to be the right ones. All of the
problems must be completed.
Relevant Reading
RAMILISON, H.A. (2006). Dioptre et miroir sphérique. Madagascar. Antanarivo
University. Unpublished course. (Give also more accessible reading lists)
Reading Summary
This course is an introduction to the study of lenses, essentials elements in any
optical system. It will describe the characteristics of a curved boundary, a brief
study of rigorous astigmatism will allow us to define the necessary conditions for
near sighted astigmatism also know as the Gauss conditions. With these conditions,
we will be able to define the key points that describe a curved boundary. We will
then establish the different conjugate relations depending on the origin’s position:
at the vertex (Descartes), at the focal point (Newton), or in the center. Under the
Gauss conditions we will then be able to define the characteristic planes like
principal planes, focal planes, which will facilitate the drawing of light rays and
therefore the possibility of solving graphs. It will also be easier to transition from
curved boundaries to curved mirrors since the only requirement wi