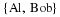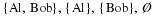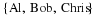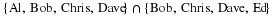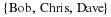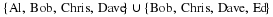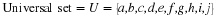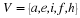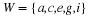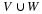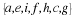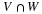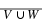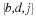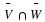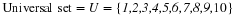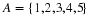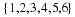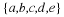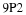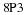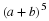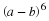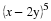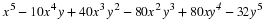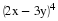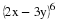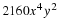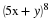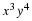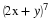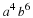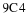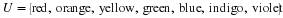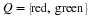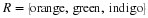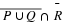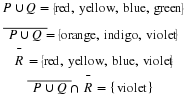Determine whether the games are strictly determined. If the games are strictly determined, find the optimal strategies for each player and the value of the game.
The game is strictly determined. Optimal strategy for the row player is to always play row 1 and never row 2. In other words, his strategy is
. The optimal strategy for the column player is to always to play column 1 and never play column 2. We write it as
. When both players play their optimal strategy, the value of the game is 1.
The game has no saddle point, therefore, it is not strictly determined.
The game is strictly determined. The optimal strategy for the row player is to always play row 4, and never play any other row. We write his strategy as
. The column player’s strategy is
. The value of the game is 2.
Two players play a game which involves holding out one or two fingers simultaneously. If the sum of the fingers is more than 2, Player II pays Player I the sum of the fingers; otherwise, Player I pays Player II the sum of the fingers.
Write a payoff matrix for Player I.
Find the optimal strategies for each player and the value of the game.
A mayor of a large city is thinking of running for re-election, but does not know who his opponent is going to be. It is now time for him to take a stand for or against abortion. If he comes out against abortion rights and his opponent is for abortion, he will increase his chances of winning by 10%. But if he is against abortion and so is his opponent, he gains only 5%. On the other hand, if he is for abortion and his opponent against, he decreases his chance by 8%, and if he is for abortion and so is his opponent, he decreases his chance by 12%.
Write a payoff matrix for the mayor.
Find the optimal strategies for the mayor and his opponent.
The optimal strategy for the mayor is
and for his opponent is
. In other words, both candidates should oppose abortion rights.
A man accused of a crime is not sure whether anybody saw him do it. He needs to make a choice of pleading innocent or pleading guilty to a lesser charge. If he pleads innocent and nobody comes forth, he goes free. However, if a witness comes forth, the man will be sentenced to 10 years in prison. On the other hand, if he pleads guilty to a lesser charge and nobody comes forth, he gets a sentence of one year and if a witness comes forth, he gets a sentence of 3 years.
Write a payoff matrix for the accused.
If you were his attorney, what strategy would you advise?
Determine the optimal strategies for both the row player and the column player, and find the value of the game.
The optimal strategy for the row player is
. The optimal strategy for the column player is
. The value of the game is 0.
Optimal strategy for the row player is
. The optimal strategy for the column player is
. The value of the game is
16/7.
Find the expected payoff for the given game matrix G if the row player plays strategy R, and column player plays strategy C.
Two players play a game which involves holding out one or two fingers simultaneously. If the sum of the fingers is even, Player II pays Player I the sum of the fingers. If the sum of the fingers is odd, Player I pays Player II the sum of the fingers.
Write a payoff matrix for Player I.
Find the optimal strategies for both the row player and the column player, and the value of the game.
Optimal strategy for the row player is
. The optimal strategy for the column player is
. The value of the game is
−1/12.
In December 1995, President Clinton ordered the first of 20,000 U. S. troops to be sent into Bosnia-Herzegovina as a peace keeping force. Unfortunately, the heavy fog made visibility very poor at the Tuzla airfield, and at the same time increased the threat of sniper attacks from the Serbian forces. U. S. Air Force Col. Neal Patton, and Lt. Col. Sid Kooyman, the advance specialists, had two choices: either to send in the troops by air with the difficulties already described or by road thus exposing the troops to ambush by the Serbian forces. The Serbian army, with its limited resources, had a choice of deploying its forces near the airport or along the road route.
If the U. S. lands its troops on the airfield in the fog while the Serbs are concentrating on the road route, the payoff for U. S. is 20 points. But if the U. S. lands its troops on the airfield, and Serbians are there hiding in the fog, U. S. wins only 5 points. On the other hand, if U. S. transports its troops by road and avoids Serbs its payoff is 35 points, but if U. S. meets Serb resistance on the road route, it loses 50 points.
Write a payoff matrix for the game.
If you were Air Force Col. Neal Patton's advisor, what advice would you give him?
Reduce the payoff matrix by dominance. Find the optimal strategy for each player and the value of the game.
Determine whether the games are strictly determined. If the games are strictly determined, find the optimal strategies for each player and the value of the game.
,
,
value=3
,
,
v=−1
,
,
value=3
,
v=3
Two players play a game which involves holding out a nickel or a dime simultaneously. If the sum of the coins is more than 10 cents, Player I gets both the coins; otherwise, Player II gets both the coins.
Write a payoff matrix for Player I.
Find the optimal strategies for each player and the value of the game.
,
,
value=5 cents
Lacy's department store is thinking of having a major sale in the month of February, but does not know if its competitor store Hordstrom's is also planning one. If Lacy's has a sale and Hordstrom's does not, Lacy's sales go up by 30%, but if both stores have a sale simultaneously, Lacy's sales go up by only 5%. On the other hand, if Lacy's does not have a sale and Hordstrom's does, Lacy's loses 5% of its sales to Hordstrom's, and if neither of the stores has a sale, Lacy's experiences no gain in sales.
Write a payoff matrix for Lacy's.
Find the optimal strategies for both stores.
,
value=5%
Mr. Halsey has a choice of three investments: Investment A, Investment B, and Investment C. If the economy booms, then Investment A yields 14% return, Investment B returns 8%, and Investment C 11%. If the economy grows moderately, then Investment A yields 12% return, Investment B returns 11%, and Investment C 11%. If the economy experiences a recession, then Investment A yields a 6% return, Investment B returns 9%, and Investment C 10%.
Write a payoff matrix for Mr. Halsey.
What would you advise him?
,
or
,
,
value=.11
Mr. Thaggert is trying to decide whether to invest in stocks or in CD's(Certificate of dep