Many people like to write linear functions in the form f( x) = b+ mx because it corresponds to the way we tend to speak: “The output starts at b and increases at a rate
of m.”
For this reason alone we will use the f( x) = b+ mx form for many of the examples, but remember they are equivalent and can be written correctly both ways.
Slope and Increasing/Decreasing
m is the constant rate of change of the function (also called slope). The slope
determines if the function is an increasing function or a decreasing function.
f ( x) = b + mx is an increasing function if m > 0
f ( x) = b + mx is a decreasing function if m < 0
If m = 0, the rate of change zero, and the function f ( x) = b + 0 x = b is just a horizontal line passing through the point (0, b), neither increasing nor decreasing.
Example 1
Marcus currently owns 200 songs in his iTunes collection. Every month, he adds 15
new songs. Write a formula for the number of songs, N, in his iTunes collection as a
function of the number of months, m. How many songs will he own in a year?
The initial value for this function is 200, since he currently owns 200 songs, so
N( )
0 = 200 . The number of songs increases by 15 songs per month, so the rate of
change is 15 songs per month. With this information, we can write the formula:
N( m) = 200 + m
15 .
N(m) is an increasing linear function.
With this formula we can predict how many songs he will have in 1 year (12 months):
N
)
12
(
= 200 +
)
12
(
15
= 200 +180 = 380 . Marcus will have 380 songs in 12 months.
102 Chapter 2
Try it Now
1. If you earn $30,000 per year and you spend $29,000 per year write an equation for
the amount of money you save after y years, if you start with nothing.
“The most important thing, spend less than you earn! 2 ”
Calculating Rate of Change
Given two values for the input, x a
n
d x , and two corresponding values for the output,
1
2
y a n
d y , or a set of points, ( x y and ( x y , if we wish to find a linear function
2
, 2)
1
, 1)
1
2
that contains both points we can calculate the rate of change, m:
change i out
n put
y
∆
y − y
2
1
m =
=
=
change i i
n nput
x
∆
x − x
2
1
Rate of change of a linear function is also called the slope of the line.
Note in function notation, y = f x and y = f x , so we could equivalently write 2
( 2)
1
( 1)
f ( x − f x
2 )
( 1)
m =
x − x
2
1
Example 2
The population of a city increased from 23,400 to 27,800 between 2002 and 2006. Find
the rate of change of the population during this time span.
The rate of change will relate the change in population to the change in time. The
population increased by 27800 − 23400 = 4400 people over the 4 year time interval. To
find the rate of change, the number of people per year the population changed by:
4400 people
people
= 1100
= 1100 people per year
4 years
year
Notice that we knew the population was increasing, so we would expect our value for m
to be positive. This is a quick way to check to see if your value is reasonable.
Example 3
The pressure, P, in pounds per square inch (PSI) on a diver depends upon their depth
below the water surface, d, in feet, following the equation P( d) = .
14 696 + 434
.
0
d .
Interpret the components of this function.
2 http://www.thesimpledollar.com/2009/06/19/rule-1-spend-less-than-you-earn/
Section 2.1 Linear Functions
103
The rate of change, or slope, 0.434 would have units output pressure PSI
=
=
. This
input
depth
ft
tells us the pressure on the diver increases by 0.434 PSI for each foot their depth
increases.
The initial value, 14.696, will have the same units as the output, so this tells us that at a
depth of 0 feet, the pressure on the diver will be 14.696 PSI.
Example 4
If f ( x) is a linear function, f )
3
( = 2
− , and f )
8
( = 1, find the rate of change.
f )3( = 2− tells us that the input 3 corresponds with the output -2, and f )8( =1 tells us that the input 8 corresponds with the output 1. To find the rate of change, we divide the
change in output by the change in input:
change i out
n put 1− (− )
2
3
m =
=
= . If desired we could also write this as m = 0.6
change i i
n nput
8 − 3
5
Note that it is not important which pair of values comes first in the subtractions so long
as the first output value used corresponds with the first input value used.
Try it Now
2. Given the two points (2, 3) and (0, 4), find the rate of change. Is this function
increasing or decreasing?
We can now find the rate of change given two input-output pairs, and can write an
equation for a linear function once we have the rate of change and initial value. If we
have two input-output pairs and they do not include the initial value of the function, then
we will have to solve for it.
Example 5
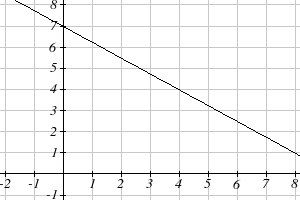
Write an equation for the linear function
graphed to the right.
Looking at the graph, we might notice that it
passes through the points (0, 7) and (4, 4).
From the first value, we know the initial value
of the function is b = 7, so in this case we will
only need to calculate the rate of change:
104 Chapter 2
4 − 7 − 3
m =
=
4 − 0
4
This allows us to write the equation:
f ( x
3
) = 7 − x
4
Example 6
If f ( x) is a linear function, f )
3
( = 2
− , and f )
8
( = 1, find an equation for the function.
In example 3, we computed the rate of change to be
3
m = . In this case, we do not
5
know the initial value f ( )
0 , so we will have to solve for it. Using the rate of change,
we know the equation will have the form f ( x = b 3
)
+ x . Since we know the value of
5
the function when x = 3, we can evaluate the function at 3.
3
f )
3
( = b +
)
3
(
Since we know that f )
3
( = 2
− , we can substitute on the left side
5
3
− 2 = b +
)
3
(
This leaves us with an equation we can solve for the initial value
5
9 −19
b = 2
− − =
5
5
Combining this with the value for the rate of change, we can now write a formula for
this function:
f
−
( x
19 3
) =
+ x
5
5
Example 7
Working as an insurance salesperson, Ilya earns a base salary and a commission on each
new policy, so Ilya’s weekly income, I, depends on the number of new policies, n, he
sells during the week. Last week he sold 3 new policies, and earned $760 for the week.
The week before, he sold 5 new policies, and earned $920. Find an equation for I(n),
and interpret the meaning of the components of the equation.
The given information gives us two input-output pairs: (3,760) and (5,920). We start
by finding the rate of change.
920 − 760 160
m =
=
= 80
5 − 3
2
Section 2.1 Linear Functions
105
Keeping track of units can help us interpret this quantity. Income increased by $160
when the number of policies increased by 2, so the rate of change is $80 per policy; Ilya
earns a commission of $80 for each policy sold during the week.
We can then solve for the initial value
I( n) = b + 80 n
then when n = 3, I(3) = 760 , giving
760 = b +
)
3
(
80
this allows us to solve for b
b = 760 −
)
3
(
80
= 520
This value is the starting value for the function. This is Ilya’s income when n = 0,
which means no new policies are sold. We can interpret this as Ilya’s base salary for
the week, which does not depend upon the number of policies sold.
Writing the final equation:
I( n) = 520 + 80 n
Our final interpretation is: Ilya’s base salary is $520 per week and he earns an
additional $80 commission for each policy sold each week.
Flashback
Looking at Example 7:
Determine the independent and dependent variables.
What is a reasonable domain and range?
Is this function one-to-one?
Try it Now
3. The balance in your college payment account, C, is a function of the number of
quarters, q, you attend. Interpret the function C(a) = 20000 – 4000 q in words. How many quarters of college can you pay for until this account is empty?
Example 8
Given the table below write a linear equation that represents the table values
w, number of 0
2
4
6
weeks
P(w), number 1000
1080
1160
1240
of rats
We can see from the table that the initial value of rats is 1000 so in the linear format
P( )
w = b + mw, b = 1000.
Rather than solving for m, we can notice from the table that the population goes up by
80 for every 2 weeks that pass. This rate is consistent from week 0, to week 2, 4, and 6.
106 Chapter 2
The rate of change is 80 rats per 2 weeks. This can be simplified to 40 rats per week and
we can write
P( )
w = b + mw as P( w) = 1000 + 40 w
If you didn’t notice this from the table you could still solve for the slope using any two
points from the table. For example, using (2, 1080) and (6, 1240),
1240 1080 160
m
−
=
=
= 40 rats per week
6 − 2
4
Important Topics of this Section
Definition of Modeling
Definition of a linear function
Structure of a linear function
Increasing & Decreasing functions
Finding the vertical intercept (0, b)
Finding the slope/rate of change, m
Interpreting linear functions
Try it Now Answers
1. S( y) =
000
,
30
y −
000
,
29
y = 1000 y $1000 is saved each year.
−
2.
4 3
1
1
m =
=
= − ; Decreasing because m < 0
0 − 2 − 2
2
3. Your College account starts with $20,000 in it and you withdraw $4,000 each quarter
(or your account contains $20,000 and decreases by $4000 each quarter.) You can pay
for 5 quarters before the money in this account is gone.
Flashback Answers
n (number of policies sold) is the independent variable
I(n) (weekly income as a function of policies sold) is the dependent variable.
A reasonable domain is (0, 15)*
A reasonable range is ($540, $1740)*
*answers may vary given reasoning is stated; 15 is an arbitrary upper limit based on
selling 3 policies per day in a 5 day work week and $1740 corresponds with the domain.
Yes this function is one-to-one
Section 2.1 Linear Functions
107
Section 2.1 Exercises
1. A town's population has been growing linearly. In 2003, the population was 45,000,
and the population has been growing by 1700 people each year. Write an
equation, P( t)
,
for the population t years after 2003.
2. A town's population has been growing linearly. In 2005, the population was 69,000,
and the population has been growing by 2500 people each year. Write an
equation, P( t)
,
for the population t years after 2005.
3. Sonya is currently 10 miles from home, and is walking further away at 2 miles per
hour. Write an equation for her distance from home t hours from now.
4. A boat is 100 miles away from the marina, sailing directly towards it at 10 miles per
hour. Write an equation for the distance of the boat from the marina after t hours.
5. Timmy goes to the fair with $40. Each ride costs $2. How much money will he have
left after riding n rides?
6. At noon, a barista notices she has $20 in her tip jar. If she makes an average of $0.50
from each customer, how much will she have in her tip jar if she serves n more
customers during her shift?
Determine if each function is increasing or decreasing
7. f ( x) = 4 x + 3
8. g ( x) = 5 x + 6
9. a( x) = 5 − 2 x
10. b( x) = 8 − 3 x
11. h( x) = 2
− x + 4
12. k ( x) = 4
− x +1
13. j ( x) 1
= x − 3
14. p( x) 1
= x − 5
2
4
15. n( x)
1
= − x − 2
16. m( x)
3
= − x + 3
3
8
Find the slope of the line that passes through the two given points
17. (2, 4) and (4, 10)
18. (1, 5) and (4, 11)
19. (-1,4) and (5, 2)
20. (-2, 8) and (4, 6)
21. (6,11) and (-4,3)
22. (9,10) and (-6,-12)
108 Chapter 2
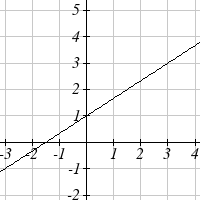
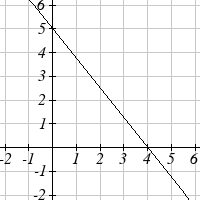
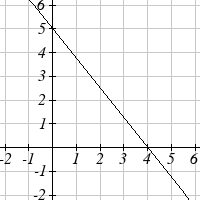
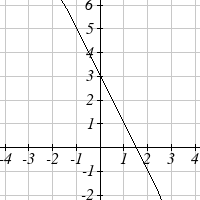
Find the slope of the lines graphed
23.
24.
25. Sonya is walking home from a friend’s house. After 2 minutes she is 1.4 miles from
home. Twelve minutes after leaving, she is 0.9 miles from home. What is her rate?
26. A gym membership with two personal training sessions costs $125, while gym
membership with 5 personal training sessions costs $260. What is the rate for
personal training sessions?
27. A city's population in the year 1960 was 287,500. In 1989 the population was
275,900. Compute the slope of the population growth (or decline) and make a
statement about the population rate of change in people per year.
28. A city's population in the year 1958 was 2,113,000. In 1991 the population was
2,099,800. Compute the slope of the population growth (or decline) and make a
statement about the population rate of change in people per year.
29. A phone company charges for service according to the formula: C( n) = 24 + 0.1 n , where n is the number of minutes talked, and C( n) is the monthly charge, in dollars.
Find and interpret the rate of change and initial value.
30. A phone company charges for service according to the formula: C( n) = 26 + 0.04 n , where n is the number of minutes talked, and C( n) is the monthly charge, in dollars.
Find and interpret the rate of change and initial value.
31. Terry is skiing down a steep hill. Terry's elevation, E( t) , in feet after t seconds is given by E( t) = 3000 − 70 t . Write a complete sentence describing Terry’s starting elevation and how it is changing over time.
Section 2.1 Linear Functions
109
32. Maria is climbing a mountain. Maria's elevation, E( t) , in feet after t minutes is given by E ( t) =1 200 + 40 t . Write a complete sentence describing Maria’s starting
elevation and how it is changing over time.
Given each set of information, find a linear equation satisfying the conditions, if possible
33. f ( 5)
− = 4
− , and f (5) = 2
34. f ( 1)
− = 4 , and f (5) =1
35. Passes through (2, 4) and (4, 10)
36. Passes through (1, 5) and (4, 11)
37. Passes through (-1,4) and (5, 2)
38. Passes through (-2, 8) and (4, 6)
39. x intercept at (-2, 0) and y intercept at (0, -3)
40. x intercept at (-5, 0) and y intercept at (0, 4)
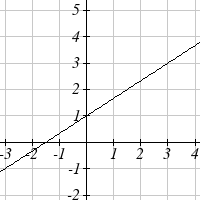
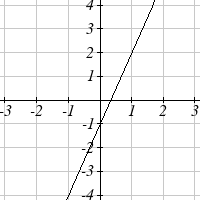
Find an equation for the function graphed
41.
42.
43.










