TEASER - 15
TELESCOPIC EFFECT
On a local scale, compare an aerial photograph (seen from top) of a mountain with contour map of same mountain or an aerial photograph of a tall building with contour map of same building.
The shape of the mountain, or the tall building would be exactly same in both aerial photograph and contour map:
(a) Falls
(b) True
THE EXPLANATION:
The aerial photograph is a method of creating 2D image of relief of an undulating surface by means of variations of light and shade. The contour maps are systematized form of showing same variations of light and shade by means of lines connecting equal elevation or depth.
The most important thing in determining shape and size of 3D objects in contour map or aerial photograph is whether relative position of each point, with respect to each other, have been maintained taking into account the element of height, length and width.
The contour map (especially on a local scale) maintains the relative position of each points with respect to each other as seen in ground at all level. However, even though aerial photographs do not hide anything, it deforms the feature and distorts the relative position of points seen in ground. The nearer objects appear bigger and farther one becomes smaller telescopically. The objects seen within the ‘view finder’ have to be adjusted for depth element because photographs or image in 2D formats lack depth.
One scale would not be applicable for all the objects seen within same photo-frame and shall vary according to depth. Therefore shape of object get distorted proportionately with depth in an aerial photograph.
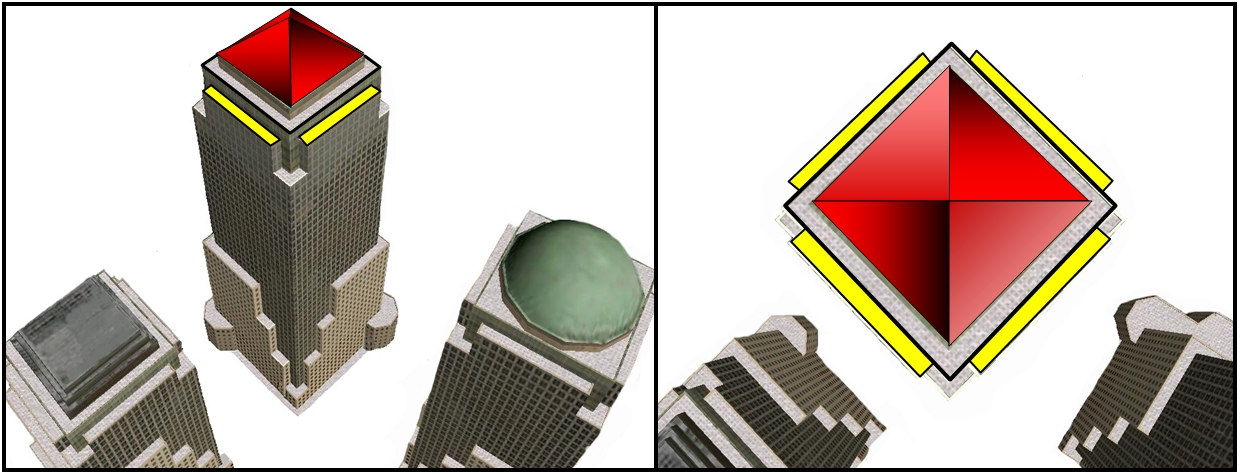
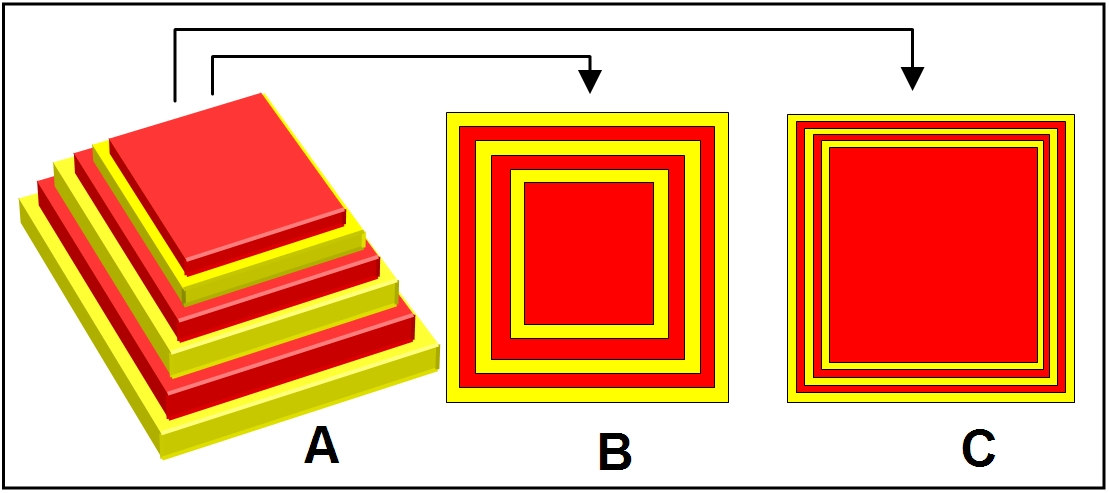
Take for example a pyramid shaped structure with staircase type slopes as shown in figure below (A).
• In the map view (contour map) of the structure (B), the relationship between each step with respect to their size and position remain constant irrespective of relative distance of each step from top to bottom. The ratio of an area between top step and bottom step would remain same as would be in reality.
• Single map scale would be applicable for each step, irrespective of their depth or height from reference level (datum level) on which map is made.
• In an aerial photograph (C), taken from very close range from top, the step closer to the viewer would appear bigger than the step away from viewer (towards bottom). The ratio between top and bottom steps with respect to size and position would not be same as would be in reality. The ratio would depend on how close the photograph is taken from.
• Single scale would not be applicable for all steps in the aerial photograph (C). Each step shall have to have its own scale depending upon its depth from the level on which photograph is referenced.
SHADED CONTOURS (B) & PHOTOGRPH (C) OF A PYRAMID (A) SEEN FROM TOP
• In an extreme case (D), only the top stair would be visible in the aerial photograph and all below would be hidden behind. It may appear like an inverted pyramid, like a tall building seen from above (E).
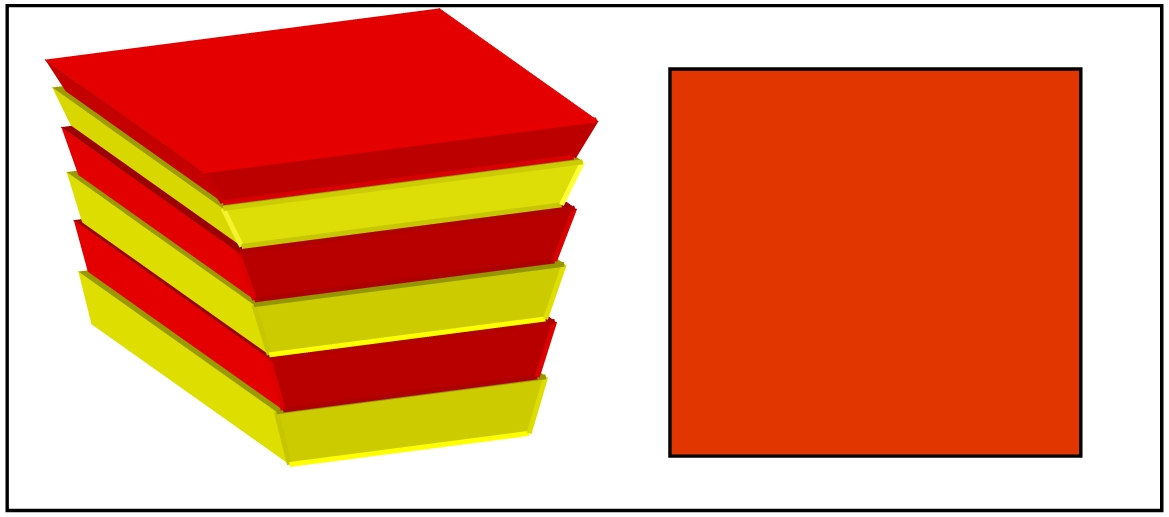
D: A PYRAMID SEEN FROM TOP AT CLOSE RANGE
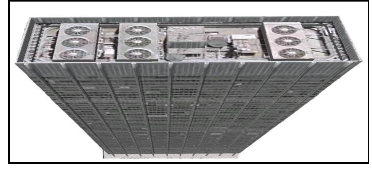
E; TALL BUILDING SEEN FROM ABOVE ROOF IS MUCH BIGGER THAN BASE
********







