10. The Use of Time-Forwarding to Place Satellites in Orbit

Richard Lighthouse
Creative Commons 4.0 International License; 2020 by Richard Lighthouse.
CC BY 4.0
Please acknowledge source. RLighthouse.com

ISBN: 9781311927323
Revision –2a-- 29 July 2014
Houston, Texas, U.S.A.
12 February 2014
WARNING: The NSA has likely placed electronic tracking tags in this document. Please consider printing for distribution.
Abstract
Satellites are becoming smaller and less expensive, mostly due to the advances in electronics, while the launch costs remain prohibitive for mass commercial use. Launch costs for a typical telecommunications satellite are around $120MM USD. This short technical paper proposes a method for placing a 500 kg (1100 lb) satellite in orbit for less than $2 USD. The avoided-cost savings for the US, could exceed $50 Billion USD over twenty years. This technology is based on a derivative of time travel. Each reader must comprehend that our universe literally blinks off and on, many billions of times every second.
Introduction
Readers are encouraged to review references [1] and [2] prior to this paper, as this short paper will make more sense.
The launch vehicle and launch services for a typical telecommunications satellite cost around $120MM USD. [3] This is only the cost of the launch vehicle and launch services. The cost of the satellite is separate. According to reference [3] in typical USD ($ millions):
Satellite Manufacture $150MM
Satellite Launch $120MM
Launch Insurance $ 20MM
In-Orbit Insurance $ 20MM
Satellite Operations $ 15MM
Total $325MM
In 2011, DARPA estimated that some satellites were costing up to $30,000 per pound ($66,100 per kg) to place in orbit, so there is a real incentive to find lower-cost methods for placing satellites in orbit.[4]
Virgin Galactic has stated for the media they intend to offer some launch services (under 500 lbs) at less than $10 MM per satellite.[5] And while this is certainly more attractive financially, what if we could greatly reduce these costs? Satellites are becoming smaller and less expensive, mostly due to the advances in electronics, while the launch costs remain prohibitive for mass commercial use.
Proposed in this paper is one practical use for electrical time travel technology, which has been described in previous papers.[6] The cost to place a 500 kg satellite (including the booster engine) into orbit can be done at a cost of approximately $2 USD worth of electricity. Two US dollars. The explanation follows:
Our earth is orbiting the sun at 67,000 miles per hour (108,000 km per hour). If you are located at the equator, you are also spinning at approximately 1000 miles per hour (1610 km per hour).
If we use time-forwarding to place a satellite in orbit, the satellite will not maintain our inertial reference frame. If, for example, it is sent 20 minutes into the future, this becomes a position about 22,000 miles out in space, in our terms. (There are other secondary effects which we will neglect for the purposes of this discussion.)
From this position, a satellite would immediately descend in free-fall towards the earth, accelerating as it descends. In effect, this free energy becomes the first propellant stage for the vehicle. As the satellite descends, a typical Holman orbit transfer can be initiated which will circularize and stabilize the orbit.
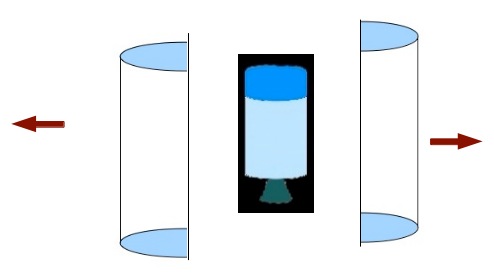
Figure 1. The Satellite is designed with a special aluminum shroud that is discarded in space. The shroud protects the satellite electronics from the large electric charge that forwards the vehicle in time.
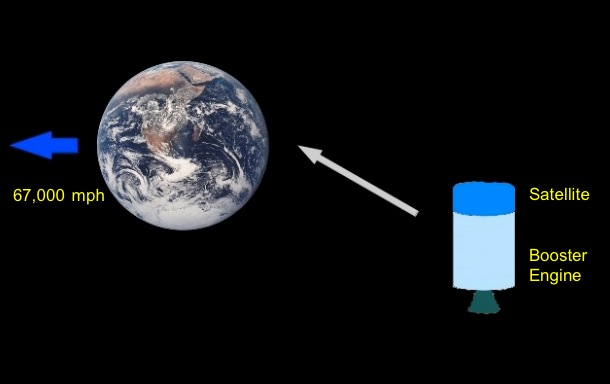
Figure 2. After being time-forwarded, the Satellite will immediately begin a free-fall. The booster engine is then used to circularize the orbit.
Conclusions
This paper has described a novel and low-cost method for placing satellites in orbit. The cost is so low, that individual consumers may be able to use the technology in the near future. For example, an expendable CubeSat could be placed in space without a typical propulsion system, and although it would have a short life, it could collect data and/or take pictures for about 45 minutes (free fall). The cost to place it in space would be less than $1 USD of electricity.
An interesting question to ask is: How much does the US spend on launch vehicles, launch services, and launch insurance each year? Now multiply that number times 20 years – that's the avoided cost in using this technology. An initial estimate probably places this value at over $50 Billion USD. [7]
This document is a living document. The author reserves the right to make corrections and changes. The mathematics used in this paper are not intended to be precise or all-inclusive. They are intended to represent the significant cost savings that may be achievable by utilizing the described technology. Capital costs for the time machine equipment are assumed to be small relative to the long-term operational costs (electricity).
References
1. Richard Lighthouse, “Mathematical Solution Unifying the Four Fundamental Forces in Nature,” smashwords.com, 2013.
2. Richard Lighthouse, “Time Travel: An Approximate Mathematical Solution,” smashwords.com, 2013.
3. quora.com; retrieved 11 Feb 2014.
4. DARPA, 2011; darpa.mil/NewsEvents/Releases/2011/11/10.aspx
5. Virgin Galactic; virgin.com/richard-branson/introducing-launcherone
6. Richard Lighthouse, “Prototype Design for a Time Machine,” smashwords.com, 2013.
7. spacedaily.com;
http://www.spacedaily.com/reports/More_Than_1200_Satellites_To_Be_Launched_Over_The_Next_10_Years_999.html; 7 September 2010.
APPENDIX
500 kg (1100 lb) satellite, forwarded in time 20 minutes.
Part 1
We will assume a value of 3.1 m/sec per year for dc/dt [2]
For our satellite to move forward in time 20 minutes -
dc/dt = 3.1 m/s/yr *1/8760 hr/yr *1 hr/3600 sec * 20 min* 60 sec/min
dc/dt =.000118 m/sec
Part 2
The Volt*Amp*sec requirements are:
V*A*sec/2 = 2c * m * dc
V*A*sec = 2 * 2c * m * dc [units are m/s * kg * m/s]
= 4 * c * 500 * .000118
= 70,727,292 volt*amps*sec
= 70,272 kVA sec [units are kvolts * amps * sec]
= 19.65 kW.hr
@ 10 cents per KW.hr ---
This equals about $2 USD.
= = = = = =
Additional Jumps
It is possible that making one or more small time jumps during or after the orbital burn, will help to minimize the size and duration of the booster engine.
A computer simulation to optimize this design process is recommended, for a given mass and orbit.






