Bid to Win: A Guide to Pursuit, Capture, and Management of Unprecedented or High Technology Projects by Evin Stump - HTML preview

Chapter 18—The efficient bidding frontier

Unfortunately, life is not always so simple that in competing for new work we will quickly settle on a single technical baseline, a single programmatic approach, and a single corresponding bid amount. What frequently happens in practice is that a firm will generate two, three, or perhaps even more alternative approaches and will want to compare them in one or more meaningful ways.

There are many ways to compare alternative approaches to the same project. There is, however, an interesting and useful way most people never consider, and that is to think of the project as a family of alternative investment opportunities. This requires application of the principles of time value of money. There is more than one way to do that, but most economists hold that the best of these is the net present value approach. That is what we advocate here, but with a twist. The twist is adapted from the work of Harry Markowitz, a famous economist and winner of the John von Neumann Theory Prize and the Nobel Memorial Prize in Economics.

The approach is widely used in investment analysis, where risk and return are both evaluated and used to make investment decisions, such as decisions to buy or sell stocks. The approach has been little used in product / market decisions, such as decisions to add or drop product lines, or, as we are interested in here, which of several options to bid competitively. But it can have value in that context.

If you have analyzed each alternative approach to a particular bidding opportunity with both the Best Bid and the Best Plan models, you will have, for each of them, a win probability and series of values of net income (revenues minus costs) for each month of the project.

Let the net income in month m be Gm. Also, let the firm’s cost of capital rate be r. Then, the net present value of the cash flows is given by the usual formula:

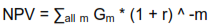
For each alternative, we can convert NPV to an expectation by multiplying it by its win probability, W:

ENPV = NPV * W

In investment analysis, it is also usual to consider “risk.” This is not the so-called “private” risk associated with a project, such as the probability that the product will fail a critical test. It is the so-called “public” risk associated with the investment, typically as measured by its volatility in trading. The usual measure is the standard deviation of its price over time.

That type of volatility is not appropriate to a decision as to which of several bidding options to go with, because there is no time history. But there is another type of volatility that can be of interest. Recall now the following paragraph from the preceding chapter:

“Because of the nature of the labor force in the advanced contracts business, a primary goal of most re-planning is to achieve stabilization of the labor force insofar as possible. Indeed, many advanced contracts firms treat this stabilization as a goal secondary only to the overall profitability of the firm. In some cases, immediate profit gains are sacrificed for the sake of stability, on grounds that stability will enhance longer term profits. Planned schedules are sometimes modified for the same reason.”

If we want to pursue stabilization in bidding, we can do so by minimizing the volatility among the alternatives we are looking at. We can arrive at an expectation of volatility using the following formula, which is based on the standard deviation.

EVOL = NPV sqrt(W (1 – W))

At first glance, we can always minimize volatility by bidding the alternative that has the highest win probability. But this ignores the quality of the projects won and could lead to poor results over the long run. ENPV is a reasonable proxy for quality, so how do we choose between values of quality and values of volatility?

Here is where Harry Markowitz’s concept of an efficient frontier comes into play. From here we will proceed with a numerical example to make clear what is being done. Suppose that a hypothetical firm TEK-R-US is looking to bid a new project and has developed four alternative approaches with the following results:

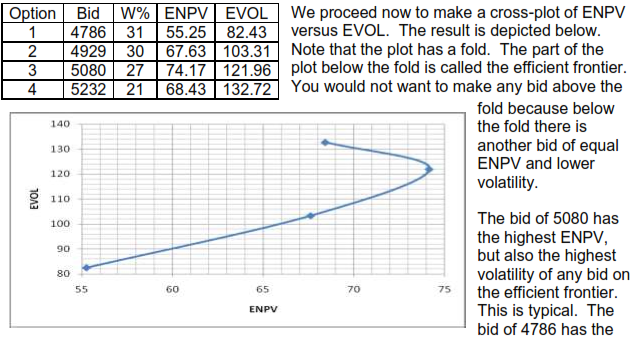
lowest ENPV, but also the lowest volatility. The efficient frontier approach has two benefits:

• It clearly shows certain options we would most likely never want to pursue.
• Of the options we might want to pursue on the efficient frontier, it clearly shows the tradeoff between risk and return, with “risk” being understood not as risk associated with possible problems during performance, but as a risk to bidder financial stability that is assumed from bidding high in a competitive environment.

A natural question to ask is what is the best point below the fold? The efficient frontier view does not answer this question. However, the Best Bid model does.