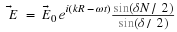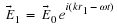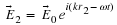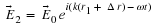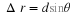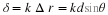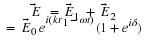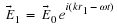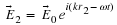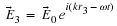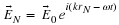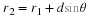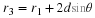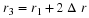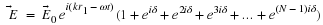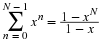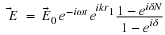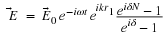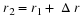One of the more difficult concepts we encounter in physics is the notion of a field. However it is an extremely useful concept. A scalar field is a map over some space of scalar values. That is it is a map of values with no direction. A simple example of a scalar field is a map of the temperature distribution in a room. In this course the most important example is the electromagnetic potential field. Below are a few examples of graphical representations of one particular scalar field.

Figure 4.1. 

A vector field can be considered a map of vectors over some space. . For example if one were to show wind vectors on a weather map; that would be a vector field. The electric field surrounding a charge is a vector field (were is the potential around the charge is a scalar field).

The flux of a vector field through a closed surface is the average outward normal component of the vector times the area of the surfaceflux = (average normal component ) ⋅ (surface area)

Three vector fields are shown below. Which represents the electric field eminating from a positive point charge in the middle? (Note that vectors of similar magnitude are colored similarly in these plots)

Figure 4.2. 

Assume we have measured the temperature in a room along an axis x . If we wanted to find the temperature change as we move to postion ( x + Δ x ) then from the fundamental definition of a derivative we know that is:

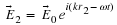
We can easily extend this concept to 3 dimensions At position ( x , y , z ) there is a temperature T ( x , y , z ) . Suppose we then want to find the temperature at . Then we can use: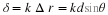

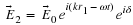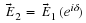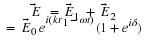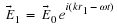
We could define a vector and then say so let's define an operator Then we can write 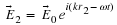is a vector operator that can be used in other situations involving scalars and vectors. It is often named "del" or "nabla". Operating on a scalar field with this operator is called taking the "gradient" of the field.

We could also operate on a vector field with del. There are two different ways to do this, by taking the dot and the cross products. To operate on a vector field by taking its dot product with del is referred to as taking the divergence. ie. 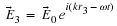where is some vector field and f is the resulting scalar field.

Similarly one could take the cross product: where 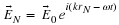is the resulting vector f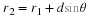ield. This is referred to as taking the curl of a field.

These operations, Gradient, Divergence and Curl are of fundamental importance. They have been presented above as operations using some newly defined operator but they in fact have deep physical significance. When using these operators to express Maxwell's equations in differential form, the meaning of these operations will hopefully become more clear. Gradient is the easiest to understand, it can be thought of as a three dimensional slope.

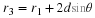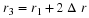
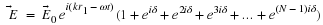
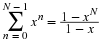
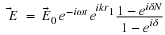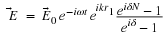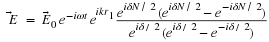Having defined these operations we can go on to second derivative type things Note that ∇² occurs so often that is has its own name, Laplacian Here is an example of taking a divergence that will be extremely useful. If and and

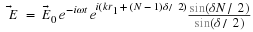then lets find (for 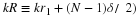is a constant vector)

Consider the following volume enclosed by a surface we will call S .

Figure 4.3. 

Now we will embed S in a vector field:

Figure 4.4. 

We will cut the the object into two volumes that are enclosed by surfaces we will call S₁ and S₂.

Figure 4.5. 

Again we embed it in the same vector field.

Figure 4.6. 

It is clear that flux through S₁ + S₂ is equal to flux through S . This is because the flux through one side of the plane is exactly opposite to the flux through the other side of the plane:

Figure 4.7. 

So we see that We could subdivide the surface as much as we want and so for n subdivisions the integral becomes:

What is .? We can subdivide the volume into a bunch of little cubes:

Figure 4.8. 

To first order (which is all that matters since we will take the limit of a small volume) the field at a point at the bottom of the box is where we have assumed the middle of the bottom of the box is the point . Through the top of the box you get Through the top and bottom surfaces you get Flux Top - Flux bottom

Which is

Likewise you get the same result in the other dimensionsHence

or

So in the limit that Δ V_i → 0 and n → ∞

This result is intimately connected to the fundamental definition of the divergence which is where the integral is taken over the surface enclosing the volume V . The divergence is the flux out of a volume, per unit volume, in the limit of an infinitely small volume. By our proof of Gauss' theorem, we have shown that the del operator acting on a vector field captures this definition.

This is derived in a similar fashion to Gauss' theorem, except now, instead of considering a volume and taking a surface integral, we consider a surface and take a line integral around the edge of the surface. In this case the surface does not enclose a volume. A good picture that describes what is being done is figure 2.23 in Berkeley Physics Course Volume 2. (Unfortunately it is copyrighted, and so it can not be shown on the web - If somebody reading this can provide some suitable drawings I would incorporate them with an acknowledgment). The following figures are not as good as in the book, but will have to do for now. We have some surface with a vector field passing through it:

Figure 4.9. 

We can take the line integral around the edge of the surface and evaluate that. We can also slice the surface into two parts

Figure 4.10. 

The line integral along the common edge will have opposite signs for each half and so the sum of the two individual line integrals will equal the line integral of the complete surface. We can subdivide as much as we want and we will always have:Since we can subdivide as much as we want we can break the surface down into a collection of tiny squares, each of which lies in the { x , y } , { y , z } or { x , z } planes.

Consider asquare in the x y plane and lets find the line integral Let us find the line integral of . First consider the x component at the center of the bottom of the square and then at the center of the top So multiplying by Δ x and subtracting the top and bottom to get the F_x contribution to the line integral gives Minus sign comes about from the integration direction (if at the top F_x is more positive this results in a negative contribution)Similarly in y we get the contribution because F_y is more positive in the right you get a positive contribution

So for this simple example which is just or if we define such that it has the area Δ x Δ y and a direction perpendicular to the xy plane it is We have shown the above is true for a square in the { x , y } plane. Similarly we would look at the other possible planes and in { xz } plane would get which is